सम्मिश्र तल: Difference between revisions
(image added) |
(added content) |
||
| Line 4: | Line 4: | ||
[[File:सम्मिश्र तल.jpg|thumb|चित्र-सम्मिश्र तल ]] | [[File:सम्मिश्र तल.jpg|thumb|चित्र-सम्मिश्र तल ]] | ||
जटिल समतल को कभी-कभी आर्गैंड समतल या गॉस समतल कहा जाता है। | जटिल समतल को कभी-कभी आर्गैंड समतल या गॉस समतल कहा जाता है। | ||
Real and Imaginary make Complex | |||
A Complex Number is a combination of a Real Number and an Imaginary Number: | |||
A Real Number is the type of number we use every day. | |||
Examples: 12.38, 2, 0, -2000 | |||
When we square a Real Number we get a positive (or zero) result: | |||
What can we square to get -1? | |||
22 = 2 x 2 = 4 | |||
12 = 1 x 1 = 1 | |||
02 = 0x0 = 0 | |||
?2 = -1 | |||
Squaring -1 does not work because multiplying negatives gives a positive : (-1) × (-1) = +1, and no other Real Number works either. | |||
So it seems that mathematics is incomplete ... | |||
... but we can fill the gap by imagining there is a number that, when multiplied by itself, gives -1 | |||
(call it i for imaginary): | |||
¡2 = -1 | |||
An Imaginary Number, when squared gives a negative result | |||
imaginary2 | |||
negative | |||
Examples: 5i, -3.6i, i/2, 500i | |||
And together: | |||
A Complex Number is a combination of a Real Number and an Imaginary Number | |||
Examples: 3.6 + 4i, −0.02 + 1.2i, 25 – 0.3i, 0 + 2i | |||
Putting a Complex Number on a Plane | |||
You may be familiar with the number line : | |||
-10 -9 -8 -7 -6 -5 -4 -3 -2 -1 0 1 2 3 4 5 6 7 8 9 10 | |||
But where do we put a complex number like 3+4i ? | |||
Let's have the real number line go left-right as usual, and have the imaginary number line go up-and-down: | |||
We can then plot a complex number like 3 + 4i : | |||
• 3 units along (the real axis), | |||
⚫ and 4 units up (the imaginary axis). | |||
And here is 4 - 2i : | |||
• 4 units along (the real axis), | |||
• | |||
and 2 units down (the imaginary axis). | |||
[[Category:सम्मिश्र संख्याएँ और द्विघातीय समीकरण]] | [[Category:सम्मिश्र संख्याएँ और द्विघातीय समीकरण]] | ||
[[Category:कक्षा-11]][[Category:गणित]] | [[Category:कक्षा-11]][[Category:गणित]] | ||
Revision as of 19:37, 28 October 2024
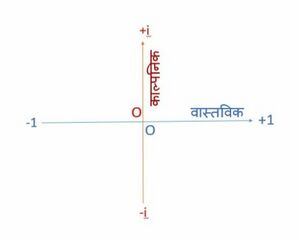
गणित में, सम्मिश्र तल एक ऐसा तल है जो सम्मिश्र संख्याओं से निर्मित होता है। इसमें कार्टेशियन निर्देशांक प्रणाली होती है, जिसमें क्षैतिज -अक्ष को वास्तविक अक्ष कहा जाता है और यह वास्तविक संख्याओं को दर्शाता है, जबकि ऊर्ध्वाधर -अक्ष को काल्पनिक अक्ष कहा जाता है और यह काल्पनिक संख्याओं का प्रतिनिधित्व करता है। सम्मिश्र तल के माध्यम से सम्मिश्र संख्याओं की ज्यामितीय व्याख्या संभव होती है।
इसके अतिरिक्त सम्मिश्र संख्याएँ सदिश की तरह जोड़ती हैं। दो सम्मिश्र संख्याओं का गुणन ध्रुवीय निर्देशांक में सरलता से व्यक्त किया जा सकता है: गुणनफल का परिमाण (या मापांक) दोनों संख्याओं के परिमाणों का गुणनफल होता है, और गुणनफल का कोण (या तर्क) दोनों संख्याओं के कोणों का योग होता है। विशेष रूप से, मापांक 1 वाली किसी सम्मिश्र संख्या से गुणा करने पर यह एक घूर्णन की तरह कार्य करती है।
जटिल समतल को कभी-कभी आर्गैंड समतल या गॉस समतल कहा जाता है।
Real and Imaginary make Complex
A Complex Number is a combination of a Real Number and an Imaginary Number:
A Real Number is the type of number we use every day.
Examples: 12.38, 2, 0, -2000
When we square a Real Number we get a positive (or zero) result:
What can we square to get -1?
22 = 2 x 2 = 4
12 = 1 x 1 = 1
02 = 0x0 = 0
?2 = -1
Squaring -1 does not work because multiplying negatives gives a positive : (-1) × (-1) = +1, and no other Real Number works either.
So it seems that mathematics is incomplete ...
... but we can fill the gap by imagining there is a number that, when multiplied by itself, gives -1
(call it i for imaginary):
¡2 = -1
An Imaginary Number, when squared gives a negative result
imaginary2
negative
Examples: 5i, -3.6i, i/2, 500i
And together:
A Complex Number is a combination of a Real Number and an Imaginary Number
Examples: 3.6 + 4i, −0.02 + 1.2i, 25 – 0.3i, 0 + 2i
Putting a Complex Number on a Plane
You may be familiar with the number line :
-10 -9 -8 -7 -6 -5 -4 -3 -2 -1 0 1 2 3 4 5 6 7 8 9 10
But where do we put a complex number like 3+4i ?
Let's have the real number line go left-right as usual, and have the imaginary number line go up-and-down:
We can then plot a complex number like 3 + 4i :
• 3 units along (the real axis),
⚫ and 4 units up (the imaginary axis).
And here is 4 - 2i :
• 4 units along (the real axis),
•
and 2 units down (the imaginary axis).