मध्य-बिंदु प्रमेय

ज्यामिति के क्षेत्र में त्रिभुजों के गुणों से संबंधित महत्वपूर्ण प्रमेयों में से एक को मध्य-बिंदु प्रमेय कहा जाता है।

मध्य-बिंदु प्रमेय के सिद्धांत का उपयोग निर्देशांक ज्यामिति में किया जाता है, जिसमें कहा गया है कि रेखाखंड का मध्यबिंदु अंत बिंदुओं का औसत होता है। इस प्रमेय का उपयोग करके समीकरण को हल करने के लिए 'x' और 'y' निर्देशांक ज्ञात होना चाहिए। मध्य-बिंदु प्रमेय कलन और बीजगणित के क्षेत्र में भी उपयोगी है।

मध्य-बिंदु प्रमेय कथन

मध्यबिंदु प्रमेय कहता है कि "किसी त्रिभुज में उसकी किन्हीं दो भुजाओं के मध्यबिंदु को मिलाने वाला रेखाखंड उसकी तीसरी भुजा के समांतर कहलाता है और तीसरी भुजा की लंबाई का आधा भी होता है।"

चित्र-1

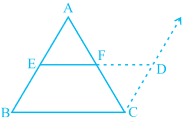
चित्र-1 में दर्शाए गए त्रिभुज में $E$ और $F$ त्रिभुज की दो भुजाओं के मध्यबिंदु हैं

$EF\parallel BC$ ,$EF={\frac {1}{2}}BC$ and $\angle AEF=\angle ABC$

इसके साथ, हम निम्नलिखित प्रमेयों पर पहुँचते हैं

प्रमेय 1: त्रिभुज की दो भुजाओं के मध्य-बिंदुओं को मिलाने वाला रेखाखंड तीसरी भुजा के समांतर होता है।

प्रमेय 2: त्रिभुज की एक भुजा के मध्य-बिंदु से होकर दूसरी भुजा के समांतर खींची गई रेखा तीसरी भुजा को समद्विभाजित करती है।

Converse of Midpoint Theorem

Statement: The converse of midpoint theorem states that "the line drawn through the midpoint of one side of a triangle that is parallel to another side will bisect the third side". We prove the converse of mid point theorem by contradiction.

Proof of Mid Point Theorem Converse

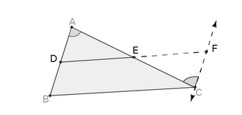
Consider a triangle $ABC$, and let $D$ be the midpoint of $AB$. A line through $D$ parallel to $BC$ meets $BC$ at $E$, as shown in the Fig. 2 below:.

Fig. 2

Given: In $\triangle ABC$, $D$ is the midpoint of $AB$ and $DE\parallel BC$.

To Prove: $E$ is the midpoint of $AC$ (i.e.,$AE=CE$)

Construction: Through $C$, draw a line parallel to $AB$ that meets the extended $DE$ at $F$

Proof of Converse of Midpoint Theorem
1. $BCFD$ is a parallelogram	$DE\parallel BC$ (given) and $BD\parallel CF$ (by construction)
2. $BD=CF$	Opposite sides of a parallelogram are equal
3.$AD=BD$	D is the midpoint of AB (given)
4. $AD=CF$	from 2 and 3
Compare $\triangle AED$ with $\triangle CEF$:
5. $\angle DAE=\angle ECF$	Alternative angles
6. $\angle DEA=\angle FEC$	Vertically opposite angles
7.$\triangle AED\cong \triangle CEF$	By AAS criterion (using 4, 5, and 6)
8. $AE=CE$	By CPCTC

This completes the proof of the converse midpoint theorem.