लम्ब वृत्तीय शंकु का आयतन

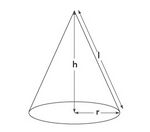
The volume of a cone defines the space or the capacity of the cone. A cone is a three-dimensional geometric shape having a circular base that tapers from a flat base to a point called apex or vertex. A cone is formed by a set of line segments, half-lines or lines connecting a common point, the apex, to all the points on a base that is in a plane that does not contain the apex.

A cone can be seen as a set of non-congruent circular disks that are stacked on one another such that the ratio of the radius of adjacent disks remains constant.

Volume of a Cone = ${\frac {1}{3}}\pi r^{2}h$

where $r$ is the base radius and $h$ is the height of the cone.

Examples

1.The height and the slant height of a cone are $21$ cm and $28$ cm respectively.

Find the volume of the cone.

Solution:

From $l^{2}=r^{2}+h^{2}$

$r={\sqrt {l^{2}-h^{2}}}$

$r={\sqrt {28^{2}-21^{2}}}={\sqrt {784-441}}={\sqrt {343}}={\sqrt {49\times 7}}=7{\sqrt {7}}$ cm

Volume of the cone = ${\frac {1}{3}}\pi r^{2}h$

=${\frac {1}{3}}\times {\frac {22}{7}}\times (7{\sqrt {7}})^{2}\times 21=7546$ cm³