एक वृत्त के चाप द्वारा अंतरित कोण: Difference between revisions
No edit summary |
(content modified) |
||
| (2 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
हमे ज्ञात है कि वृत्त के व्यास के अतिरिक्त किसी जीवा के अंतिम बिंदु उसे दो चापों में विभाजित करते हैं, जिन्हें दीर्घ चाप और लघु चाप कहते हैं। इस लेख में हम वृत्त के चाप द्वारा अंतरित कोण से संबंधित प्रमेय और उसके पूर्ण स्पष्टीकरण के साथ उसके प्रमाण पर चर्चा करेंगे। | |||
== | == एक वृत्त के चाप द्वारा अंतरित कोण – प्रमेय एवं प्रमाण == | ||
'''प्रमेय:''' | |||
एक चाप द्वारा केंद्र पर बनाया गया कोण वृत्त के शेष भाग पर किसी भी बिंदु पर बनाए गए कोण का दोगुना होता है। | |||
''' | '''प्रमाण:''' | ||
केंद्र <math>O</math> वाले एक वृत्त पर विचार करें। यहाँ वृत्त का चाप <math>PQ</math> केंद्र <math>O</math> पर कोण <math>\angle POQ</math> तथा वृत्त के शेष भाग पर स्थित बिंदु <math>A</math> पर कोण <math>\angle PAQ</math> अंतरित करता है। | |||
प्रमाण करने हेतु : <math>\angle POQ = 2 \angle PAQ</math> | |||
इसे प्रमाणित करने के लिए, <math>AO</math> को जोड़ें और इसे बिंदु <math>B</math> तक विस्तारित करें | |||
इस प्रमेय को सिद्ध करते समय दो सामान्य स्थितियाँ हैं। | |||
''' | '''स्थिति 1:''' | ||
[[File:Circle-3.jpg|alt=Fig. 1|none|thumb|150x150px| | [[File:Circle-3.jpg|alt=Fig. 1|none|thumb|150x150px|चित्र -1]] | ||
त्रिभुज <math>APO</math> पर विचार करें | |||
यहाँ, <math>OA=OP</math> (त्रिज्या) | |||
चूँकि समान भुजाओं के विपरीत कोण समान होते हैं, | |||
<math>\angle OPA = \angle OAP .... (1)</math> | <math>\angle OPA = \angle OAP .... (1)</math> | ||
इसके अतिरिक्त, बाह्य कोण गुण (बाह्य कोण आंतरिक विपरीत कोणों का योग है) का उपयोग करते हुए, | |||
हम लिख सकते हैं, | |||
<math>\angle BOP = \angle OAP + \angle OPA</math> | <math>\angle BOP = \angle OAP + \angle OPA</math> | ||
<math>(1)</math> का उपयोग करके | |||
<math>\angle BOP = \angle OAP + \angle OAP</math> | <math>\angle BOP = \angle OAP + \angle OAP</math> | ||
| Line 38: | Line 38: | ||
<math>\angle BOP = 2 \angle OAP .... (2)</math> | <math>\angle BOP = 2 \angle OAP .... (2)</math> | ||
इसी प्रकार, एक अन्य त्रिभुज <math>AQO</math> पर विचार करें , | |||
<math>OA=OQ</math> ( | <math>OA=OQ</math> (त्रिज्या) | ||
चूँकि समान भुजाओं के विपरीत कोण समान होते हैं, | |||
<math>\angle OQA = \angle OAQ .... (3)</math> | <math>\angle OQA = \angle OAQ .... (3)</math> | ||
इसी प्रकार, बाह्य कोण गुण का उपयोग करके, हम <math>\angle BOQ = \angle OAQ + \angle OQA</math> प्राप्त करते हैं | |||
<math>\angle BOQ = \angle OAQ + \angle OAQ</math> ( | <math>\angle BOQ = \angle OAQ + \angle OAQ</math> (<math>(3)</math>का उपयोग करते हुए) | ||
<math>\angle BOQ = 2 \angle OAQ .... (4)</math> | <math>\angle BOQ = 2 \angle OAQ .... (4)</math> | ||
<math>(2)</math> और <math>(4)</math> को जोड़ने पर हमें प्राप्त होता है | |||
<math>\angle BOP +\angle BOQ =2\angle OAP +2\angle OAQ</math> | <math>\angle BOP +\angle BOQ =2\angle OAP +2\angle OAQ</math> | ||
| Line 60: | Line 60: | ||
<math>\angle POQ =2\angle PAQ</math> | <math>\angle POQ =2\angle PAQ</math> | ||
अतः, स्थिति (1) सिद्ध होती है। | |||
''' | '''स्थिति 2:''' | ||
[[File:Circle-4.jpg|alt=Fig. 2|none|thumb|150x150px| | [[File:Circle-4.jpg|alt=Fig. 2|none|thumb|150x150px|चित्र -2]] | ||
इस स्थिति में <math>\angle POQ =2\angle PAQ</math> को प्रमाणित करने के लिए, हम स्थिति (1) के समान ही चरणों का पालन कर सकते हैं। लेकिन (2) और (4) को जोड़ते समय हमें नीचे दिए गए चरणों का पालन करना होगा। | |||
<math>\angle BOP +\angle BOQ =2\angle OAP +2\angle OAQ</math> | <math>\angle BOP +\angle BOQ =2\angle OAP +2\angle OAQ</math> | ||
प्रतिवर्ती कोण <math>\angle POQ =2(\angle OAP +\angle OAQ)</math> (चूँकि,<math>PQ</math> एक दीर्घ चाप है) | |||
प्रतिवर्ती कोण <math>\angle POQ =2\angle PAQ</math>. | |||
अतः सिद्ध हुआ। | |||
[[Category:वृत्त]][[Category:कक्षा-9]][[Category:गणित]] | [[Category:वृत्त]][[Category:कक्षा-9]][[Category:गणित]] | ||
Latest revision as of 19:23, 17 September 2024
हमे ज्ञात है कि वृत्त के व्यास के अतिरिक्त किसी जीवा के अंतिम बिंदु उसे दो चापों में विभाजित करते हैं, जिन्हें दीर्घ चाप और लघु चाप कहते हैं। इस लेख में हम वृत्त के चाप द्वारा अंतरित कोण से संबंधित प्रमेय और उसके पूर्ण स्पष्टीकरण के साथ उसके प्रमाण पर चर्चा करेंगे।
एक वृत्त के चाप द्वारा अंतरित कोण – प्रमेय एवं प्रमाण
प्रमेय:
एक चाप द्वारा केंद्र पर बनाया गया कोण वृत्त के शेष भाग पर किसी भी बिंदु पर बनाए गए कोण का दोगुना होता है।
प्रमाण:
केंद्र वाले एक वृत्त पर विचार करें। यहाँ वृत्त का चाप केंद्र पर कोण तथा वृत्त के शेष भाग पर स्थित बिंदु पर कोण अंतरित करता है।
प्रमाण करने हेतु :
इसे प्रमाणित करने के लिए, को जोड़ें और इसे बिंदु तक विस्तारित करें
इस प्रमेय को सिद्ध करते समय दो सामान्य स्थितियाँ हैं।
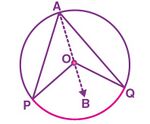
स्थिति 1:
त्रिभुज पर विचार करें
यहाँ, (त्रिज्या)
चूँकि समान भुजाओं के विपरीत कोण समान होते हैं,
इसके अतिरिक्त, बाह्य कोण गुण (बाह्य कोण आंतरिक विपरीत कोणों का योग है) का उपयोग करते हुए,
हम लिख सकते हैं,
का उपयोग करके
इसी प्रकार, एक अन्य त्रिभुज पर विचार करें ,
(त्रिज्या)
चूँकि समान भुजाओं के विपरीत कोण समान होते हैं,
इसी प्रकार, बाह्य कोण गुण का उपयोग करके, हम प्राप्त करते हैं
(का उपयोग करते हुए)
और को जोड़ने पर हमें प्राप्त होता है
अतः, स्थिति (1) सिद्ध होती है।
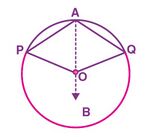
स्थिति 2:
इस स्थिति में को प्रमाणित करने के लिए, हम स्थिति (1) के समान ही चरणों का पालन कर सकते हैं। लेकिन (2) और (4) को जोड़ते समय हमें नीचे दिए गए चरणों का पालन करना होगा।
प्रतिवर्ती कोण (चूँकि, एक दीर्घ चाप है)
प्रतिवर्ती कोण .
अतः सिद्ध हुआ।