एक ही रेखा के समानांतर रेखाएँ

Fig. 1 - Transversal Line

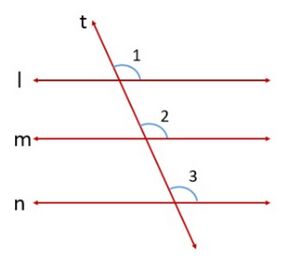
If two lines are parallel to the same line, will they be parallel to each other? Let us verify.

In the fig.1 line $m||$ line $l$ and line $n||$ line $l$ .

Let us draw a line $t$ transversal for the lines $l,m,n$

We know that line $m||$ line $l$ and line $n||$ line $l$ .

Hence $\angle 1=\angle 2$ and $\angle 1=\angle 3$ (Corresponding angles axiom)

But $\angle 2=\angle 3$ as they are corresponding angles

Therefore, we can say that line $m||$ line $n$ (Converse of corresponding angles axiom)

This result can be stated in the form of the following theorem:

Theorem 1: Lines which are parallel to the same line are parallel to each other.

Example

From the given figure, $AB||CD$, $CD||EF$, $EA\perp AB$ and $\angle BEF=55^{\circ }$. Find the values of $x,y,z$.

Fig. 2

Solution:

Given that $AB||CD$, $CD||EF$, $EA\perp AB$ and $\angle BEF=55^{\circ }$

Therefore, $y+55^{\circ }=180^{\circ }$ (Interior angles on the same side of transversal $ED$)

Hence, $y=180^{\circ }-55^{\circ }=125^{\circ }$

By using the corresponding angles axiom, $AB||CD$, we can say that $x=y$.

Therefore, the value of $x=125^{\circ }$

Since, $AB||CD$ and $CD||EF$, therefore $AB||EF$.

So, we can write: $\angle FEA+\angle EAB=180^{\circ }$(Interior angles on the same side of transversal $EA$)

$55^{\circ }+z+90^{\circ }=180^{\circ }$

$z=180^{\circ }-90^{\circ }-55^{\circ }=35^{\circ }$

Therefore, the values of $x,y,z$ are $125^{\circ },125^{\circ },35^{\circ }$ respectively.

चित्र -1 अनुप्रस्थ रेखा

यदि दो रेखाएँ एक ही रेखा के समानान्तर हों तो क्या वे एक-दूसरे के समानान्तर होंगी? आइए सत्यापित करें।

चित्र-1 में रेखा $m||$ रेखा $l$ और रेखा $n||$ रेखा $l$।

आइए हम रेखाओं $l,m,n$ के लिए एक रेखा $t$ अनुप्रस्थ रेखा खींचें

हम जानते हैं कि रेखा $m||$ रेखा $l$ और रेखा $n||$ रेखा $l$ है।

अतः $\angle 1=\angle 2$ और $\angle 1=\angle 3$ (संगत कोण अभिगृहीत)

परंतु $\angle 2=\angle 3$ क्योंकि वे संगत कोण हैं

अतः, हम कह सकते हैं कि रेखा $m||$ रेखा $n$ (संगत कोण अभिगृहीत का विलोम)

इस परिणाम को निम्नलिखित प्रमेय के रूप में बताया जा सकता है:

प्रमेय 1: वे रेखाएँ जो एक ही रेखा के समानान्तर होती हैं, एक दूसरे के समानान्तर होती हैं।