चक्रीय चतुर्भुज
चक्रीय चतुर्भुज
चक्रीय चतुर्भुज एक वृत्त में अंकित चार भुजाओं वाला बहुभुज होता है। इसमें दी गई भुजाओं की लंबाई के साथ अधिकतम संभव क्षेत्रफल होता है। दूसरे शब्दों में, एक वृत्त में अंकित चतुर्भुज उन भुजाओं की लंबाई के साथ अधिकतम संभव क्षेत्र को दर्शाता है।
चक्रीय चतुर्भुज की परिभाषा
चक्रीय चतुर्भुज का अर्थ है एक चतुर्भुज जो एक वृत्त में अंकित होता है। इसका मतलब है कि एक वृत्त है जो चतुर्भुज के सभी चार शीर्षों से होकर गुजरता है। शीर्षों को चक्रीय कहा जाता है। वृत्त के केंद्र को परिकेंद्र के रूप में जाना जाता है और वृत्त की त्रिज्या को परित्रिज्या के रूप में जाना जाता है।
शब्द "चक्रीय" ग्रीक शब्द "कुक्लोस" से लिया गया है, जिसका अर्थ है "वृत्त" या "पहिया"। शब्द "चतुर्भुज" प्राचीन लैटिन शब्द "क्वाड्री" से लिया गया है, जिसका अर्थ है "चार भुजाएँ" या "लैटस"।
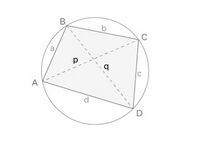
नीचे दिए गए चित्र में, एक चक्रीय चतुर्भुज है, जिसकी भुजाओं की लंबाई तथा विकर्ण हैं।
चक्रीय चतुर्भुज के गुणधर्म
The properties of a cyclic quadrilateral help us to identify this figure easily and to solve questions based on it. Some of the properties of a cyclic quadrilateral are given below:
- In a cyclic quadrilateral, all the four vertices of the quadrilateral lie on the circumference of the circle.
- The four sides of the inscribed quadrilateral are the four chords of the circle.
- The measure of an exterior angle at a vertex is equal to the opposite interior angle.
- In a cyclic quadrilateral, = sum of product of opposite sides, where are the diagonals.
- The perpendicular bisectors are always concurrent.
- The perpendicular bisectors of the four sides of the cyclic quadrilateral meet at the center O.
- The sum of a pair of opposite angles is (supplementary). Let be the four angles of an inscribed quadrilateral. Then, and.
Theorems related to Cyclic Quadrilateral are mentioned below.
Theorem 1: The sum of either pair of opposite angles of a cyclic quadrilateral is .
Theorem 2: If the sum of a pair of opposite angles of a quadrilateral is , the quadrilateral is cyclic.
चक्रीय चतुर्भुज का क्षेत्रफल
The area of a cyclic quadrilateral is where are the four sides of the quadrilateral and is the semi perimeter which can be calculated as
. Heron's formula for a triangle is also derived from this equation.
उदाहरण
1: चित्र 2 में, एक चक्रीय चतुर्भुज है जिसमें और इसके विकर्ण हैं।
यदि और हैं , ज्ञात कीजिए
हल:
(एक ही खंड में कोण)
अत:,
परंतु (चक्रीय चतुर्भुज के विपरीत कोण)
A cyclic quadrilateral is a four-sided polygon inscribed in a circle. It has the maximum area possible with the given side lengths. In other words, a quadrilateral inscribed in a circle depicts the maximum area possible with those side lengths.
एक चतुर्भुज ABCD चक्रीय कहलाता है यदि इसके चारों शीर्ष एक वृत्त पर स्थित हों (चित्र-1 देखें)।
यहाँ हमें and प्राप्त होता है।
चक्रीय चतुर्भुज से संबंधित प्रमेय नीचे उल्लिखित हैं।
प्रमेय 1: चक्रीय चतुर्भुज के विपरीत कोणों के किसी भी युग्म का योग होता है।
प्रमेय 2: यदि चतुर्भुज के विपरीत कोणों के किसी युग्म का योग है, तो चतुर्भुज चक्रीय होता है।